readers' forum
Slipped Scleral Buckle Creates Prescription Dilemma
BY SHELDON H. KREDA, OD, FAAO

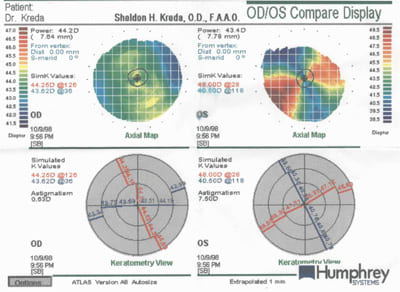
One year following surgical correction for a retinal detachment, this 45-year-old man experienced a post-op complication: his scleral buckle had dislocated anteriorly. The surgeon elected to suture the buckle into place, which deformed the sclera and created 8.00D of astigmatism. Figure 1 shows the resulting topography map.

As expected, spectacles provided poor visual acuity as well as intolerable distortion. The patient's corneal measurements were as follows:

Keratometry: 41.25 @ 125/50.00 @ 35.

Refraction: +1.50 ­8.00 x 123 20/40^-3

Restoring this patient's vision was both an obligation and a rewarding experience.

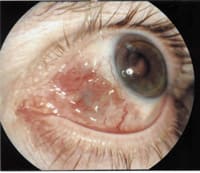
This patient was a high myope habitually corrected with soft lenses. Clearly, contact lenses were his only option to restore usable binocular vision. I selected a rigid contact lens design to avoid contact with the surgical repair adjacent to the limbus and to limit the risk of infection (Figure 2). Bitoric gas permeable lenses were necessary to achieve acceptable acuity and fit.

Figure 1. Sutures to mend a slipped scleral buckle resulted in a deformed sclera with 8.00D of astigmatism.

Calculating Bitoric Lens Parameters

To simplify the fitting of a bitoric contact lens, think of the astigmatic eye as two individual spherical eyes. For this patient, one eye is a +1.50D hyperope with a 41.25 diopter spherical cornea. The other is a ­6.50D myope with a 50.00 diopter spherical cornea.

Trial fit with a spherical lens 0.25D flatter than the flattest meridian or calculate the lens empirically. In this case, I selected a base curve of 41.00 diopters (8.23mm). Perform a sphero-cylindrical over-refraction to determine the power in each meridian.

The Flat Meridian For empirical fitting, choose a base curve 0.25D flatter than K. This will create a ­0.25D tear lens. From the refraction we know that +1.50D is needed in that meridian. If this eye was spherical, a lens of base curve 8.23mm and power of +1.75D (tear lens plus refraction) would correct his vision.

For trial fitting, order the final lens with the flat meridian equal to the base curve of the trial lens. Add the power of the trial lens to the over-refraction in that meridian to yield the final power.

	Figure 2. The smaller diameter of this rigid lens does not contact the surgical repair.

The Steep Meridian For empirical fitting, the steep base curve should be 0.75D flatter than the steep K. This will induce rocking, which promotes tear exchange (try to induce rocking along the horizontal axis, which emulates a with-the-rule cornea). In this case, the base curve would be 49.25 diopters (6.85mm). By the refraction, ­6.50D is needed in that meridian. Effectivity brings this to a ­6.00D. Add the ­0.75D tear lens due to the flatter base curve, which leaves a power of ­5.25D for optimum correction for that meridian. If you would treat this meridian as that of a spherical eye, you would order a contact lens with a base curve of 6.85mm and a power of ­5.25D.

For trial fitting, as for the flat meridian, add the power of the trial lens, the over-refraction in the steep meridian (compensate for effectivity) and the tear lens. This will yield a resultant power needed to correct vision in that meridian. Use this resultant power to calculate the power of the final lens with a base curve that is 0.75D flatter than the steep meridian. (Figure 3).

The Final Lens Just combine these two lenses to calculate the bitoric lens.

Specify the bitoric lens in this form: BC_Flat/BC_Steep, D_Flat/D_Steep (power), OAD,OZD, along with material and finishing instructions. I calculated the specifications for our patient's final lens as follows:

Base Curve = 8.23mm/6.85mm

Power = +1.50D/­5.25D.

Use your usual diameter and design parameters to complete the lens specification. We used an 8.8mm diameter with edge thinning.

Troubleshoot the final contact lens one meridian at a time using the fluorescein pattern and sphero-cylindrical over-refraction.

The final bitoric lens for our patient positioned slightly infero-nasally with good movement. The fluorescein pattern was spherical and aligned the cornea well. Acuity was 20/20^-2, and the lens restored this patient's binocular vision.

Don't shy away from clinical oddities. Helping these patients is our duty. After two years of follow-up, our patient continues to have good vision and comfort. He's thankful for the return of his eyesight.

Figure 3. Follow these steps when using trial lenses to calculate the final power of a bitoric lens.

Dr. Kreda practices in a primary care setting in Lauderhill, Florida. He's a frequent author and lecturer on practice management, contact lenses and medical optometry. You can contact him at eyerx@peoplepc.com.