prescribing for astigmatism

Interpreting SCOR Data

BY JOHN MARK JACKSON, OD, MS, FAAO

In my April column "Using an SCOR to Improve Soft Toric Fitting," I discussed how a sphero-cylinder over-refraction (SCOR) can help you refine the lens power and predict quality of vision with soft toric lenses. The only difficult part is understanding the unusual numbers you get in the SCOR.

Here's an example. If an eye has a spectacle prescription of –1.00 –3.00 x180 and we apply a soft toric lens with power –1.00 –3.00 x180, we expect the patient to see clearly if the lens doesn't rotate. The powers and cylinder axis are just what we need; our SCOR should be plano.

But what if it rotates 10 degrees to the left? Now the cylinder axes don't coincide, and the lens doesn't perfectly correct the refractive error, resulting in an SCOR of about +0.50 –1.00 x040 (Figure 1). If the lens rotates 20 degrees to the right, the SCOR would be +1.00 –2.00 x035. Why do we still have so much cylinder, and why the weird axes?

SCOR Rules

I use three rules-of-thumb to interpret these numbers. Note that they work only when the chosen diagnostic lens is close to the correct sphere and cylinder powers.

Rule 1: The spherical equivalent of the SCOR should be close to plano. In our examples above, the spherical equivalents are both plano, showing that the lens power itself is close to correct. This isn't especially helpful because we already knew that, but it does help us understand the SCOR numbers.

Rule 2: The amount of cylinder power in the SCOR increases as lens misalignment increases. For every 10 degrees of misalignment, the SCOR will have one-third of the original lens's cylinder power. The first example had 10 degrees of misalignment, which gave us one-third of our original cylinder (–1.00D) in the SCOR. In the second example we were off 20 degrees, so we got two-thirds of our cylinder (–2.00D) in the SCOR.

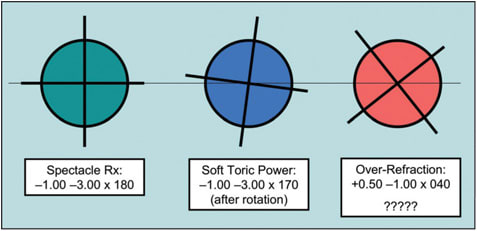
Figure 1. When the eye's axis and the lens axis are misaligned, the SCOR axis will be very oblique.

What about the oblique axes in the SCORs? For 10 degrees misalignment, intuition tells us the SCOR axis would be 005 (halfway between our spectacle axis and the lens axis). So why do we get an actual SCOR axis of 040?

The minus lens powers for this patient mean that the eye itself has too much plus power in each meridian. We're using the minus contact lens to neutralize the +4.00 –3.00 x090 error of the eye itself. This means the cylinder axes we're comparing aren't what we first thought. It turns out that the SCOR axis is rather oblique to the spectacle axis. Which brings us to…

Rule 3: When the lens misalignment is small, the SCOR axis will be quite oblique to the spectacle axis. As the misalignment increases, the SCOR axis gets closer to the spectacle axis. This counter-intuitive result is the most confusing aspect about SCORs. Don't let it worry you; just know that a really oblique SCOR is a good thing!

Putting SCOR to Practical Use

Usually, I check the SCOR numbers to see if they make sense given the amount of rotation I see when looking at the lens on the eye. I then use a SCOR calculator if I need critical refinement of the lens power. I think it helps to keep your sanity when you understand where the SCOR comes from. CLS

Dr. Jackson is an assistant professor at Southern College of Optometry where he works in the Advanced Contact Lens Service, teaches courses in contact lenses and performs clinical research.