letters to the editor

Commenting on “Using Power Profiles to Evaluate Aspheric Lenses”

The article in the January 2011 issue titled, “Using Power Profiles to Evaluate Aspheric Lenses,” by Vogt et al discusses the advantages of using wavefront sensing in characterizing the refractive designs of aspheric multifocal lenses. It also addresses one of the problems with such designs—the specification of the effective near addition that they provide. In our opinion, the data analysis presented in the manuscript (i.e. calculation of the near addition) may, perhaps, be biased toward the performance of a specific product, resulting in misleading conclusions. In an attempt to better describe the optical performance/functionality of these lenses, we first characterize their multifocal design by using the data provided in the article.

The authors used a commercially-available, purpose-built Shack-Hartmann wavefront sensor (Clear-Wave, AMO-Wavefront Sciences), which, according to the manufacturer, allows sampling from 2,800 lenslets within a 6mm diameter pupil. The derived data were used to calculate axial power profiles of multifocal lenses of two different brands: Air Optix Aqua Multifocal (Ciba Vision) and PureVision Multi-Focal (Bausch + Lomb) in a spherical power of −3.00D. These are center-near aspheric designs, intended to provide simultaneous vision correction for a range of distances by inducing negative spherical aberration (Bakaraju et al, 2010). PureVision lenses are available in two add powers (“Low” and “High”), while Air Optix Aqua Multifocal lenses are available in three add powers (“Lo,” “Med,” and “Hi”), providing refractive correction for a range of near vision demands.

Figure 1 shows replotted power profile data from Vogt et al (2011) for the range of addition lenses offered by the two brands. It is evident that the axial powers for the Low Add PureVision and Lo Add Air Optix Aqua lenses exhibit smooth (parabolic) profiles and can therefore be fitted by the following equation:

Pr = P₀ − br² [Equation 1]

where r is the radial distance from the center of the lens (in mm), P₀ is the paraxial power (at r=0), P_r is the power at radius r, and b (in D/mm²) is a constant that characterizes the power changes as a function of r.

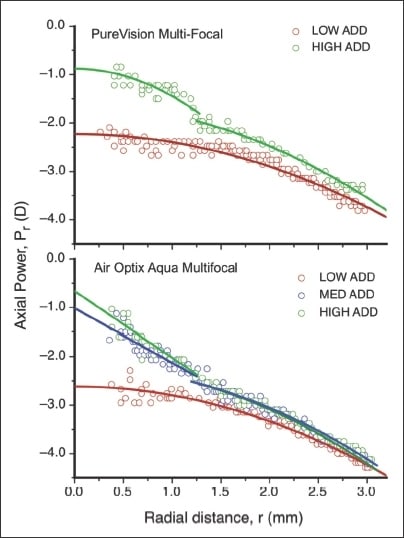
Figure 1. Plots of the axial power as a function of radial distance from the center of the lens for the range of addition lenses for PureVision Multifocal (Bausch + Lomb, upper) and Air Optix Aqua Multifocal (Ciba Vision, lower). Solid lines represent first-and second-order fitted functions. Data are replotted from Vogt et al (2011).

Using Equation 1, we find that the spherical aberration coefficients b are 0.17D/mm² and 0.18D/mm² for the “Lo Add” Air Optix Aqua and “Low Add” PureVision multifocal lenses, respectively. The only difference between the two “low add” lens types is the axial power at the center: −2.61D for the Air Optix Aqua lenses and −2.23D for the PureVision lenses, i.e. the axial power function for the PureVision lens is shifted toward less negative corrections.

The changes in axial power as a function of lens zonal radius for “Med” and “Hi/High” addition lenses (Figure 1) cannot be fitted by a single second-order function, since these lenses have bi-aspheric designs, i.e. different rates of axial power change for the central and peripheral portions. When axial power data for the peripheral zones (1.3mm < r < 3mm) are analyzed, there is no difference in the asphericity between the “Hi” and “Med” add Air Optix Aqua lenses and the “High” add of PureVision, with the spherical aberration coefficient b being 0.21D/mm² in all cases. However, the rate of change in axial power for the central 1.3mm radius differs among the three lens types. The power changes over the central region (r < 1.3mm) are best-fitted by a second-order regression (b = 0.57D/mm²) for the PureVision lens and by a linear regression of the form:

P_r = P₀ − kr [Equation 2]

where k is a constant, for the Air Optix Aqua lenses. The latter show a slighter higher slope for the “High/Hi” compared to the “Med” addition lens (k = 1.36D/mm versus 1.31D/mm). Moreover, the central PureVision power profile is shifted toward less myopic correction as compared to the Air Optix Aqua, as is also observed for the “Low/Lo” add lenses. This shift would be expected to affect the refractive correction with the two lenses but would not alter the “addition.”

Note from Figure 1 that the powers of the PureVision “Low” and “High” add lenses do not fall to the −3.00D value of the nominal distance correction until a distance of about 2.2mm and 2.5mm, respectively, from the center of the lens is reached, whereas this occurs at a distance of about 1.5mm to 2.0mm for the three Air Optix Aqua lenses. Thus, with well-centered lenses, no part of the pupil will receive the nominal −3.00D correction unless the pupil diameter exceeds about 4.4mm and 5.0mm with the PureVision lenses or 3.0mm to 4.0mm with the Air Optix Aqua lenses.

Given the power profiles of Figure 1, how do we best evaluate the “add”? Unlike traditional simultaneous-vision bifocals, there is no clearly defined “distance” and “near” power for these lenses. Instead, the gradual radial variation in power across the lens surface produces an enhanced depth-of-focus over which reasonable image quality and on-eye visual acuity can be achieved (Plakitsi & Charman, 1995; Benard et al, 2010). The through-focus nature of the image will change with the pupil diameter and, within the depth-of-focus, the “best focus” will vary with the spatial frequency spectrum of the object viewed (Plakitsi and Charman, 1997).

In spite of these difficulties, it may still be helpful to obtain some idea of the likely useful depth-of-focus. Vogt et al defined the “add” as the difference between the mean power over the central 2mm of a lens (presumably the mean value of the power profile between a 0.5mm and 1mm distance from the center of a lens) and the nominal distance correction provided by a lens (−3.00D in all cases). However, as we have seen, the Air Optix lenses tend to be more negative over much of the power profile compared to the PureVision lenses, so the assumption that the distance correction is −3.00D for both lens types tends to exaggerate the relative size of the “add” for the PureVision lenses.

We suggest a slightly different approach. It appears from Figure 1 that, in lenses in which the power profile is discontinuous, the discontinuity occurs at r = 1.3mm. We therefore evaluate the “near” correction over the central 2.6mm diameter of each lens. Further, we assume that the distance correction is the mean power of the annular zone defined by 1.3mm ≤ r ≤ 2.5mm: this mean power would, of course, become more negative if some larger pupil diameter was assumed. It can be shown that for a parabolic power profile of the type given in Equation 1, the average area-weighted power for a circular area of radius r_m (the central “near” portion) is:

P_a = P₀ − br_m²/2

For an annular area of inner and outer radii r_i and r_m this becomes:

P_a = P₀ − b(r_m²+ r_i²)/2

Finally, for a linear power profile as given in Equation 2, the average power over a circular area of radius r_m is:

P_a = P₀ − 2kr_m/3

Table 1 shows the central (near) and outer (distance) powers evaluated in this way, using r_m = 1.3mm as the boundary of the inner, “add” zone.

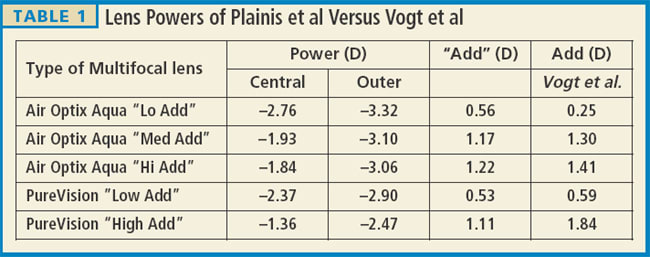
The multifocal lenses used in Vogt et al's study are labeled as −3.00D lenses, i.e. are designed to provide −3.00D correction for distance. It is evident from Table 1 that PureVision Multi-Focal lenses under-correct the refractive error for distance, instead offering better correction for near compared to the Air Optix Multifocal lenses. This under-correction is more pronounced for the “High Add” lens. The “addition,” though, does not differ between the “low” and the “high” addition types of both brands. Similarly, there seems to be little change in addition between the “Med” and “Hi” add Air Optix Multifocal lenses, but the “Hi Add” lens offers better correction for near vision. We feel that Vogt and colleagues do not make full use of the measured power profile data by calculating the “addition” from the “nominal” distance power (−3.00D) and a “measured” near power.

Power profiles are potentially of great value in indicating the characteristics of different lens designs and, ideally, should always be provided by contact lens manufacturers. However, it should be noted that wavefront analysis exhibits lower repeatability and higher variability the smaller the sampling radius or diameter (Ginis et al, 2004). This is very evident in the axial power data measured by Vogt et al, which are highly scattered at optic zone radii < 0.5mm. This is expected, since within the circular region 1mm in diameter, wave-front sensing utilizes only 75 lenses of the sensor, compared to 2,800 lenslets over a 6mm diameter region (Kollbaum et al, 2008). This may have limited the ability of the authors to collect data over the central 0.5mm radius of lenses: such data could potentially offer valuable information on contact lens designs.

We should not forget that these calculations are based on invitro measurements. A soft contact lens on the eye wraps the cornea, but several changes may occur such as lens flexure, lens dehydration and decentration, and tear lens effects, especially in cases in which the fit is not acceptable (Plainis and Charman, 1998). Moreover, performance may be limited by the inherent positive spherical aberration found in the average eye (Plakitsi and Charman, 1997; Bakaraju et al, 2010), which means that the effective add of any center-near lens tends to be reduced. Hence, although in vitro power measurements are useful, to reach safe conclusions on the efficacy of different multifocal contact lens designs, subjective and behavioral methods should be used.

Sotiris Plainis, MSc, PhD
Institute of Vision & Optics
School of Health Sciences
University of Crete, Greece
David A. Atchison, PhD
Institute of Health & Biomedical
Innovation and School of Optometry
Queensland University of Technology, Australia
W. Neil Charman, DSc
Faculty of Life Sciences
University of Manchester, UK

Dr. Vogt et al's Response

We appreciate the thoughtful analysis performed by Drs. Plainis, Atchison, and Charman, and we agree with some items they observed. In particular, we recognize the difficulty in gathering valid refractive power data at the very central portion of the contact lens with not only wavefront technology, but with conventional instruments as well (Collins et al, 1997). By the very nature of the measurements, there is an increase in standard deviation associated with an increased depth of field for small central apertures, which reduces the reliability of conventional instruments (Plakitsi and Charman, 1992).

Moreover, as Plainis et al mentioned, soft lenses conform to the eye and any multifocal design will interact with the aberrations of the eye. We agree that what happens clinically with the lens on-eye will be related to what is measured off the eye. In designing contact lenses, we use finite element modeling to predict optical properties and estimate transmitted wavefronts, which enables us to develop an optimal contact lens design. Using Hartmann-Shack wavefront sensing removes the subjectivity of individual patients, which is a known source of significant variation even in lower-order aberrations such as sphere and cylinder, and it helps researchers characterize lens designs in an unbiased nature.

Plainis et al presented an analysis of one method to determine the add power of a multifocal contact lens, and their work highlights the challenge in quantifying the add of an aspheric multifocal design. The authors used a technique whereby the power profiles were measured and then mathematical equations were developed to estimate a fit to the profiles. The challenge in fitting equations to power profiles arises when making assumptions of where an optical design is discontinuous. As the authors stated, they arbitrarily assumed the distance correction was the power of the annular zone defined by 1.3mm ≤ r ≤ 2.5mm. Without the exact lens design details from the manufacturers, this assumption adds great variability to the resulting fitting equations and the calculated add values. Any number of zones or the average power over different pupil sizes could be used to define the effective add power.

For the lens designs evaluated, it is known that add power is located in the central portion of these aspheric multifocal designs, with the center of the optic zone corrected for near vision and the power gradually changing—becoming increasingly more minus toward the periphery. The method of calculating the multifocal add power described by Vogt et al, by subtracting the average power over the central 2mm diameter from the labeled power, was selected for several reasons. First, manufacturers use labeled power to best represent the distance power that the lens will have on-eye and secondly, labeled power is the one metric that practitioners have readily available when fitting a multifocal lens. We standardized the add power measure across a 2mm zone because it represented an effective add diameter, minimized the impact of measurement variability within the central 0.25mm, and avoided impact of the transition zones in the lens geometry. To clarify an assumption made by Plainis et al, we used a high-resolution Hartman-Shack wavefront sensor from Optocraft rather than the ClearWave (AMO-Wavefront Sciences) and we only disregarded noisy data from the central 0.25mm radius.

Regardless of the method used to calculate the add power, the conclusion remains that the analysis of power profiles of the Air Optix Aqua Lo Add, Med Add, and Hi Add multifocals showed that there are effectively two distinct designs. In fact, the method presented by Plainis et al showed even less differential in add power between the Air Optix Aqua Med Add and Hi Add designs than the Vogt method did; Plainis et al calculated the Air Optix Aqua Med Add and Hi Add multifocal designs to have a difference in add of only 0.05D. This difference in add is far less than the clinical metric of 0.25D needed to differentiate designs, and therefore the Air Optix Aqua Med Add and Hi Add are considered to represent the same functional add power. Plainis et al confirmed that the PureVision Multi-Focal Low Add and High Add lenses have two distinct designs.

Plainis et al demonstrated that there are other approaches for evaluating add powers depending upon exactly which criteria are used. Both methods have demonstrated one of the fundamental points that both PureVision and Air Optix Aqua multifocal products offer two distinct functional add powers. For the reasons we have outlined above, we stand by our selection of criteria and conclusions.

For references for these letters, please visit www.clspectrum.com/references.asp and click on document #185.