SCLERAL SETTLING
What Causes Settling in Scleral Contact Lenses?
Multiple forces factor into the initial and delayed settling of scleral lenses.
By Chuck Aldridge, OD, MBA, FAAO
Scleral and mini-scleral contact lenses are now becoming more mainstream than ever. These lenses are no longer just for “sick eyes”; many patients can use them due to their wide range of prescriptions and their ability to be worn comfortably by patients suffering from contact lens-related dryness issues. As more patients are wearing these contact lenses, it is advisable for practitioners to gain a better understanding of not just how to fit them, but also of one of their idiosyncrasies known as “settling.”
With the advent of optical coherence tomography (OCT), the distance from the back surface of a scleral contact lens to the front surface of the cornea can now be accurately measured. This quantitative ability has led to the observation that this distance decreases over time to a certain degree; this has been referred to as “settling.” Not only does this settling occur shortly after application of the lens (initial settling), but it appears to continue for a period of time much longer than would be expected (delayed settling).
Therefore, the obvious questions are: What is this settling, and what forces are applicable? The purpose of this article is not to specifically answer these questions, but rather to create discussion and debate that hopefully will move us closer to a better understanding of “What causes settling in scleral contact lenses?”
Sinking
Scleral contact lenses are applied to the eye and initially ride on a layer of fluid (tear film). But, we might wonder how buoyant they are. Do they float on or within the tear film, or do they completely sink below the tear layer? To discover the answer, we need to review Archimedes’ principle, which states that the upward buoyant force that is exerted on a body immersed in a fluid, whether fully or partially submerged, is equal to the weight of the fluid that the body displaces. When calculations are applied, the results state that if the object has negative buoyancy, it will eventually sink to the bottom; if it has positive buoyancy, it will float on or within the fluid.
Even though the principle is relatively simple, the calculations are more involved and beyond the scope of this article. In the simplest terms, all scleral contact lenses, when placed in water (a similar fluid to the tear film), will eventually sink to the bottom of the container. This means that they have negative buoyancy and will sink onto the surface of the eye. This negative buoyancy means that scleral contact lenses will not float and eventually will land on the conjunctiva after application. This landing pressure on the conjunctiva is what has been traditionally been referred to as settling and comprises part of what is considered the “initial settling.”
Suction
However, before we address settling further, we need to consider suction. This is the force that actually holds the scleral lens to the eye. Without suction, the lens would not stay aligned or perform properly.
At the 2015 Global Specialty Lens Symposium, Patrick J. Caroline, FAAO, FCLSA, and Lynette Johns, OD, FAAO, theorized that suction could be the reason why scleral lenses move gradually closer to the cornea. In addition, they suggested that suction also could perhaps explain the phenomenon of delayed settling (Caroline et al, 2015). Their theory is that the cornea is gradually being drawn upward toward the back surface of the contact lens during the wear cycle.
Intuitively, anyone who has attempted to remove a tightly adhering scleral lens would completely agree with this hypothesis. The force needed to pull a scleral lens tangentially from the eye surface and break this suction force is sometimes so great that it even produces a temporary degree of ocular bulging.
Let’s examine this concept more closely. A suction cup uses negative fluid pressure of air or water to adhere to a nonporous surface, creating a partial vacuum in the process. When applied, this suction cup (a scleral lens in our case) causes the fluid behind it to be expelled to some degree past its circular edge. This loss of fluid creates decreased pressure behind the contact lens. The pressure difference between the atmosphere on the outside of the contact lens and the lower-pressure cavity inside the contact lens causes adherence or suction.
If external fluid (tears) were able to completely replace the volume of fluid lost during application, then the suction force is eliminated. But, as we know, the tear exchange under scleral lenses is minimal, causing the adherence force to be maintained throughout the wear cycle.
How great is this force? The amount of force required to detach the suction cup (contact lens) by pulling it directly away from the surface is: F = AP, where F = force, A = area of surface covered by the suction cup, and P = pressure outside of the suction cup, which is normally atmospheric pressure.
If we look at two scleral lenses that are 14.6mm and 16.0mm in diameter, respectively, we discover that the 14.6mm lens exerts 17 Newtons of force, whereas the 16.0mm lens exerts 20 Newtons of force (Table 1).
| 14.6mm Scleral Lens | 16.0mm Scleral Lens |
| 14.6mm = 7.3mm radius | 16.0mm = 8.0mm radius |
| π (.0073)2 = .000167 (Area) | π(.008)2 = .0002 (Area) |
| .000167 x 100,000Pa = 17 Newtons | .0002 x 100,000Pa = 20 Newtons |
The clinical relevance here is that the suction force created is greater for larger-diameter contact lenses. In our example above, the suction force is about 18% greater when going from a 14.6mm diameter to a 16.0mm diameter. This should lead clinicians to select the smallest scleral contact lens possible in fitting.
However, it needs to be pointed out that this force is created immediately once the suction cup (scleral contact lens) is applied. So, if the cornea is drawn toward the contact lens, it should occur very rapidly after application because this force does not increase, and it may decrease with tear exchange, during wear. Therefore, any decrease in the distance from the posterior back surface of the contact lens to the front of the cornea that occurs later in the wear cycle (delayed settling) after the initial settling phase cannot be attributed to suction.
Squeeze
What do a rubber ball and the eye have in common? They both are elastic to some degree. And if you encircle the rubber ball with your forefinger and thumb and gently squeeze the ball, it bulges slightly.
Because a scleral contact lens has a landing zone that is circumferential around the front of the eye, could there be a squeeze force that would cause the contact lens and the cornea to become closer?
Most likely, this squeeze force is not the answer. A properly fit scleral lens does not impinge the conjunctival blood flow nor create blanching. Therefore, if the pressure against the eye is not sufficient to create blanching, we could infer that the sclera is not compressed. And if the elastic sclera is not compressed, then there is insufficient force present to create squeeze of the globe, which, in turn, makes that force irrelevant.
Through this, practitioners also could imply that there is no overt compression of the sclera and that the scleral tissue does not play a role in settling. This means scleral contact lenses only affect the conjunctiva, and the conjunctiva alone is the tissue responsible for what is known as settling.
Gravity
The degree of settling also does not seem to be dependent upon the mass (weight) of the contact lens. In a patient who is standing or sitting, the mass of the contact lens is affected by gravity, which draws the contact lens down, not toward the eye. This is why a larger, bulky contact lens will often ride lower than desired.
Contact Lens/Conjunctiva Relationship
The conjunctiva is not only soft, but also elastic. This means that once a scleral contact lens sinks into the conjunctiva, it should slightly compress this tissue. Once equilibrium is reached, the distance from the scleral contact lens to the cornea should remain constant. But, we have seen that this distance decreases further after equilibrium is reached, leading to delayed settling.
Scleral lenses land on the conjunctiva; however, they do so with varying degrees of compression. Some contact lenses have a wider landing zone, while others have narrower ones. Contact lenses that have wider landing zones have a larger surface area against the conjunctiva and will not compress the conjunctiva as deeply, given a constant force. Likewise, a narrow landing zone will create more conjunctival compression.
Regardless of the lens size, the equilibrium of conjunctival compression will occur, and what is typically considered initial settling should soon be completed. However, it is not unusual for settling to continue to occur for periods of time well beyond this point (Kauffman, 2014; Caroline and André, 2012; DeNaeyer, 2014). So why does this settling continue beyond the time it takes for the conjunctiva to be compressed?
Elasticity and Deformity
Because the conjunctiva is elastic, a review of elasticity will help us understand this delayed settling. According to Hooke’s Law (named after British physicist Robert Hooke), elasticity is the property of a solid material to return to its original shape and size after the forces deforming it have been removed. Stress (the force causing this change to the surface) is proportional to strain (the change in spatial dimensions compared to its original value).
The relationship of this stress and strain is explained by a coefficient known as the elasticity modulus. There are three basic elastic moduli: 1) bulk modulus states that an object’s volume changes, but shape does not; 2) shear modulus states that rectangles become parallelograms; and 3) Young’s modulus states that objects becomes longer and thinner or shorter and fatter.
The bulk modulus (also called compression modulus) states that a force applied uniformly over the surface of an object will compress it uniformly. This changes the volume of the object without changing its shape. The stress created here is one we are all familiar with—pressure. This stress then results in volume strain.
The bulk modulus is realized during blinking. But how does the force created by the lid tension of blinking comes into play? The lid tension of blinking, in and of itself, should not be sufficient enough to cause any significant decrease in the distance from the back surface of the contact lens to the front surface of the eye. It has been estimated that the mean static upper eyelid pressure on the ocular surface is 8.0mm (± 3.4mm)—a pressure not that great compared to the 90mm peak pressure needed to deform the cornea in noncontact tonometry (Shaw et al, 2010). But even if this lid tension were sufficient to decrease the contact lens-to-cornea distance, it would occur soon after application and would not account for delayed settling.
Blinking does create a downward force onto the contact lens surface. The stress from this pressure causes some strain within the contact lens. This strain is not sufficient to change the shape of the contact lens, but it does create a force that is transferred to the eye at the point where the contact lens touches the eye, or the landing zone.
By this time, equilibrium has been reached, and the contact lens has already settled into the conjunctiva. Therefore, this added and repeated force by the lid cannot push the contact lens deeper into the conjunctiva, it can only push the contact lens edge into the adjacent conjunctiva. This leads to the consideration of the shear modulus.
Once the landing zone of the scleral lens has embedded and sunk into the conjunctiva, the edge of the contact lens can be visualized by OCT as adjacent to this tissue. Therefore, the shear modulus (also called rigidity modulus) can explain the elasticity that occurs next (Figure 1).
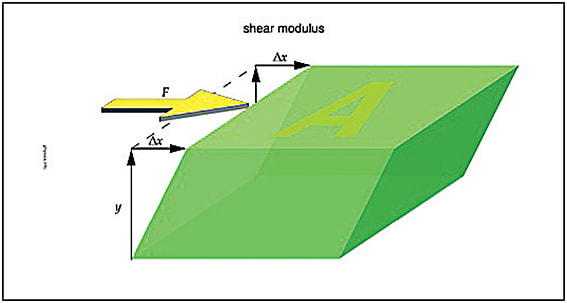
Figure 1. The shear modulus states that rectangles become parallelograms.
COURTESY OF THE PHYSICS HYPERTEXTBOOK
A force applied tangentially—or transversely or laterally—to the face of an object is shear stress. This exists when the edge of the contact lens presses against the conjunctiva. The resulting deformity is called shear strain. Any clinician who has ever manipulated the conjunctival tissue knows that it can be easily deformed, meaning that this shear modulus could be applicable to the scleral contact lens-conjunctiva relationship.
Young’s modulus (named after British scientist Thomas Young) also cannot be discounted, and it may be relevant to the conjunctiva. For example, imagine a piece of dough that is stretched. As it is stretched, it becomes longer and thinner. Likewise, if the same dough is compressed, it becomes shorter and fatter.
In Young’s modulus, it is tensile stress and tensile strain that would cause the conjunctiva to extend its length and to become thin. Then, compressive stress and compressive strain occur, which causes the conjunctiva to return to its original shape and thickness. Additionally, there are transverse contractions that occur with the axial extensions (this is known as Poisson’s ratio; named after Siméon Poisson).
If we observe the stretched dough, however, we will note that it does not completely return to its original shape; there seems to be a resulting deformity creating by this stretching. It seems that regardless of whether you subscribe to the shear modulus or Young’s modulus theories of elasticity regarding the conjunctiva, the conjunctiva does not completely return to its original shape and size and maintains some residual deformity.
While this residual deformity of the conjunctiva certainly can account for delayed settling, it does not fully explain what causes it. This concept is best understood by a discussion of viscoelasticity.
Viscoelasticity
It seems that soft tissues, such as the conjunctiva, may not necessarily follow the basic elasticity principles previously covered. Soft tissues can undergo large deformations and still have the ability to come back to their initial configuration. The stress-strain relationship of soft tissue is nonlinear, and these tissues are viscoelastic, incompressible, and usually anisotropic.
Viscoelasticity, a property of materials that exhibit both viscous and elastic traits when undergoing deformation, will be our primary focus in seeking the answer for delayed setting. Viscous materials (e.g., oil) resist shear flow and strain linearly with time when a stress is applied. Elastic materials strain when stretched, and they quickly return to their original configuration once the stress is removed.
Viscoelastic materials possess elements of both of these properties and therefore will demonstrate time-dependent strain. Generally, elasticity is the result of bond stretching along crystallographic planes in an ordered solid, and viscosity is the result of the diffusion of atoms or molecules inside an amorphous material (Meyers and Chawla, 1999). The viscoelastic properties that can be seen in soft tissues such as the conjunctiva are relaxation, creep, and hysteresis (Humphrey, 2003; Fung, 1993).
It is this tissue creep that seems to account for the residual deformation that leads to delayed settling in scleral contact lenses. Tissue creep is the elongation of tissue beyond its intrinsic extensibility, resulting from a constant load over time (Wilhelmi et al, 1998).
So, once the scleral contact lens undergoes the initial settling, the scleral lens will continue to place stress on the conjunctiva until it is elongated beyond its ability to return to its original configuration. The result is that the conjunctiva is permanently stretched further from the limbus, and that causes the landing zone of the scleral lens to also migrate further from the limbus. Once this permanent deformation occurs, the scleral lens will now settle closer to the cornea and demonstrate this delayed settling.
In Conclusion
As mentioned initially, it was not my goal to answer, “What causes settling in scleral contact lenses?” Rather, my intent was to propose ideas that hopefully will create further discussion and research on this topic that will lead us to a better understanding of this important area in scleral contact lenses. CLS
Acknowledgement: I would like to thank John Satta, a mechanical engineer, for his time in reviewing this manuscript and providing additional insight and ideas.
For references, please visit www.clspectrum.com/references and click on document #239.

| Dr. Aldridge is a graduate of Southern College of Optometry and practices in Burnsville, NC. He lectures frequently and has written numerous articles on ocular disease, contact lenses, and practice management. He is a consultant or advisor to TearLab Corporation and has received lecture or authorship honoraria from Alcon and Allergan. |